 Short Communication
Short Communication
The rT − X Family of Distributions induced by V: A New Method for Generating Continuous Distributions with Illustration to Cancer Patients Data
Clement Boateng Ampadu, Department of Biostatistics, USA.
Received Date: March 29, 2019; Published Date: April 26, 2019
Abstract
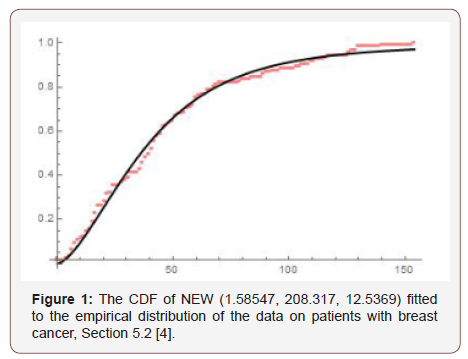
In this short note we introduce a new technique to generate continuous distributions which is partially inspired by [1] and [2]. An illustration to cancer patients data is also given, indicating the new class of distributions will be useful in modeling and forecasting data in various disciplines.
Keywords: Quantile generated probability distributions; Cancer patients data, T − X(W) family of distributions
Introduction
At first we recall the qT − X (V) framework which is inspired by [1] and [2]
Definition 3.1. Let V be any function such that the following holds:
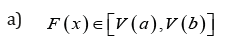
b) F (x) is differentiable and strictly increasing
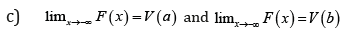
then the CDF of the qT − X (V) family induced by V is given by
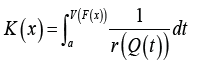
where 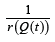 is the quantile density function of random variable
T ∈ [a,b] , for −∞ ≤ a < b ≤ ∞, and F (x) is the CDF of any random
variable X.
is the quantile density function of random variable
T ∈ [a,b] , for −∞ ≤ a < b ≤ ∞, and F (x) is the CDF of any random
variable X.
Theorem 3.2. The CDF of the qT − X family induced by V is given by
K ( x) = Q[V (F ( x))]
Proof. Follows from the previous definition and noting that 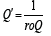
Theorem 3.3. The PDF of the qT − X family induced by V is given by
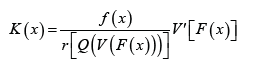
Proof.  and K is given by Theorem 3.5.2
and K is given by Theorem 3.5.2
Remark 3.4. When the support of T is[a,∞), where a ≥ 0 , we can take V as follows
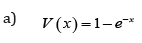
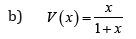
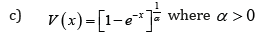 where α > 0
where α > 0
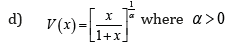 where α < 0
where α < 0
Remark 3.5. When the support of T is (−∞,∞) , we can take V as follows
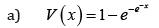
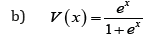
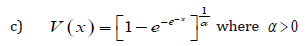 where α > 0
where α > 0
 where α > 0
where α > 0
Motivation for the New Family
Suppose a random variable T has quantile function Q, quantile density q, CDF R, and PDF r, then since
Q = R−1
The following is clear
Q(R(t )) = t and R(Q(t )) = t
If we differentiate both sides of, R(Q(t )) = t , with respect to t and solve for q(t), we get the integrand in Definition 4.1. On the other hand, if
we differentiate both sides of, Q(R(t )) = t ,with respect to t, and solve
for r(t), we can see that 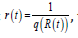 thus making this replacement to
the integrand
thus making this replacement to
the integrand
in Definition 1.1 leads to the following
Definition 4.1. Let V be any function such that the following holds:
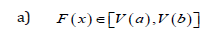
b) F (x) is differentiable and strictly increasing
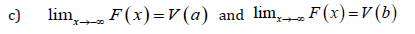
then the CDF of the rT − X family induced by V is given by
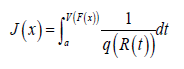
where 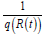 is the probability density function of random
variable T ∈[a,b] , for ] −∞ ≤ a < b < ∞, and F(x) is the CDF of any
random variable X.
is the probability density function of random
variable T ∈[a,b] , for ] −∞ ≤ a < b < ∞, and F(x) is the CDF of any
random variable X.
Notation 4.2. Ψ will denote the class of all functions V satisfying (a), (b), and (c) in the definition immediately above
The CDF of the New Family
Since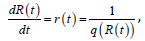 implies the following
implies the following
Theorem 5.1. The CDF of the Tr − X family induced by V is given by
J ( x) = R(V (F ( x)))
where the random variable T ∈[a,b] , for −∞ ≤ a < b < ∞, has CDF R,V ∈Ψ , and F(x) is the CDF of any random variable X.
The PDF of the New Family
By differentiating the CDF in Theorem 5.1, with respect to x, we have the following
Theorem 6.1. The PDF of the Tr − X family induced by V is given by
j ( x) = r (V (F ( x)))V′(F ( x)) f ( x)
where the random variable T ∈[a,b] , for −∞ ≤ a < b < ∞, has PDF r,V ∈Ψ, F (x) and f(x) are the CDF and PDF, respectively, of any random variable X.
Practical Significance
At first we introduce the following
Definition 7.1. A random variable K will be said to follow the new Tr − X family of distributions of type I, if the CDF can be expressed as the following integral
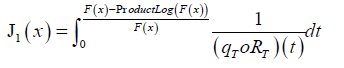
where the random variable X has CDF F(x), and the random variable T with support [0,∞) has quantile density qT and CDF RT, and Product Log [z] gives the principal solution for w in z = wew
Remark 7.2. Note that is our weight
in the above definition, and
is our weight
in the above definition, and
V−1 gives 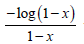 which is the weight function introduced
in [3]
which is the weight function introduced
in [3]
Since 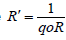 which is the weight function introduced, then the above definition implies the following
which is the weight function introduced, then the above definition implies the following
Theorem 7.3. The CDF of the new Tr − X family of distributions of type I is given by
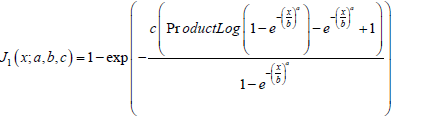
where the random variable T with support [0,1) has CDF RT, Product Log[z] gives the principal solution for w in z = wew , and the random variable X has CDF F(x)
Remark 7.4. By differentiating the CDF above, the PDF of the new Tr − X family of distributions of type I can be obtained
By assuming  , that is, X is Weibull distributed
and
, that is, X is Weibull distributed
and 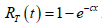 , that is, T is exponentially distributed. We get
the following from Theorem 7.3
, that is, T is exponentially distributed. We get
the following from Theorem 7.3
Theorem 7.5. The CDF of the new Exponential-Weibull family of distributions of type I is given by
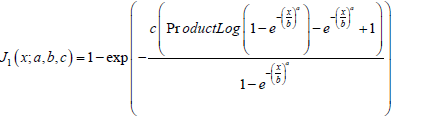
where a, b, c, x > 0, and Product Log[z] gives the principal solution for w in z = wew

Remark 7.7. The PDF of the new Exponential-Weibull family of distributions of type I can be obtained by differentiating the CDF above.
Acknowledgement
None.
Conflict of interest
No conflict of interest.
References
- Clement Ampadu (2013) Results in Distribution Theory and Its Applications Inspired by Quantile Generated Probability Distributions, Lulu.com. ISBN: 0359249957,9780359249954.
- Clement Boateng Ampadu (2018) Quantile-Generated Family of Distributions: A New Method for Generating Continuous Distributions. Fundamental Journal of Mathematics and Mathematical Sciences 9(1): 13-34.
- Zubair Ahmad, M Elgarhy, GG Hamedani (2018) A new Weibull-X family of distributions: properties, characterizations and applications. Journal of Statistical Distributions and Applications 5:5
- Girish Babu Moolath, Jayakumar K (2017) T-Transmuted X Family of Distributions, STATISTICA, anno LXXVII, n. 3.
-
Clement Boateng Ampadu. The rT-X Family of Distributions induced by V: A New Method for Generating Continuous Distributions with Illustration to Cancer Patients Data. AnnalBiostat & Biomed Appli. 2(2): 2019. ABBA.MS.ID.000531.
Quantile generated probability distributions; Cancer patients data, T − X(W) family of distributions
-

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.






