In Vivo Non-Invasive Analysis of the Mechanical Properties of Vessel Walls using Vibrational Optical Coherence Tomography

In this study we use vibrational optical coherence tomography and ultrasound to image and measure the mechanical properties of arteries and veins in vivo at rest and after running. Our results suggest that several arteries and veins are tethered together via the surrounding ECM, both laterally and longitudinally. The collagen fibers and smooth muscle cells of arterial and venous walls give rise to a mechano-vibrational peak at 140-160 Hz with a modulus of between 4 and 5 MPa. Exercise increases the arterial modulus by about 2 MPa and can influence the properties of surrounding arteries and veins via the adjacent extracellular matrix. It is concluded that lateral vascular tethering results in effective stress transfer between arteries and neighboring veins. This limits stress concentrations and transmits mechanical information between the arterial and venous sides of the vascular system. Longitudinal tethering provides mechanical coupling up and down the arterial system that conveys loading information via the wall shear stress wave that propagates through the vascular tree. In this manner local changes in external and internal mechanical loading can influence anabolic and catabolic responses in other segments of the vasculature as a result of mechanotransduction.

Keywords:ECM= Extracellular Matrix; VOCT= Vibrational Optical Coherence Tomography

Blood vessels serve as conduits for the distribution and collection of blood to and from various tissues and organs of the body. Collagen and elastic fibers, and endothelial and smooth muscle cells are the structural materials in blood vessel walls that support this function. These components are normally under tension as indicated by the contraction of arterial vessel length and diameter by about 40% when the vessel is cut [1]. Blood pressure induced strain in the human aorta is between 10% and 20% indicating that the aorta operates in the beginning part of the high strain region of the stress-strain curve [2]. Mechanical stress has been report ed to influence smooth muscle cell phenotype and other behaviors in vivo. The effect of mechanical stress on vascular smooth muscle cells leads to the production of platelet derived growth factor and mechanical stretch activated extracellular signal-regulated kinases involved in the MAP Kinase pathway [3, 4]. Specifically, steady intraluminal pressure in blood vessels activates the extracellular signal-regulated kinases, the ERK1/2 pathway [4]. Cyclic strain significantly reduces DNA synthesis in smooth muscle suggesting that mechanical loading may down-regulate proliferation of smooth muscle cells [5].

Mechanical coupling between the cellular components and collagen, elastic fibers and smooth muscle cells in the extracellular matrix (ECM) may regulate the mechanical properties of the vessel wall. A number of in vitro studies using arterial strips have been performed to assess the relative contribution of each of these components [6, 7]. The literature underscores the role of ECM in mechanochemical transduction by cells as a major factor in vessel wall mechanics [8]. This emphasizes the need to further understand how external and internal loading affects changes in the mechanical properties of arteries and veins in vivo.

Numerous studies have examined the mechanical properties of blood vessels in relation to the histological composition in vitro [9- 11]. The results of uniaxial incremental stress-strain testing studies indicate that the elastic modulus of arteries is 1.9 ± 1.4 MPa while that reported for veins is higher (6.9 ± 4.6 MPa) at high strains [9, 10]. The same study also reported a low strain modulus of 0.033 ± 0.012 MPa for arteries and 0.21 ± 0.22 MPa for veins in an animal study [10]. Lang, et al. [12] reported that the incremental elastic modulus of aorta in vivo is between 3 MPa at 22 years old and about 5 MPa at 50. Recent studies suggest that the modulus of several arteries in vivo is between 3.6 and 4.9 MPa [13-16] which is stiffer than the dermal collagen network (stiffness of 2 to 3 MPa) in the neighboring skin [13-16]. In most studies the vascular tissue tested in vitro is preconditioned before performing mechanical testing in order to give reproducible results. Preconditioning of the vascular tissue effectively changes the viscous component of the blood vessel mechanics [10]. Aside from this, the properties of vessels, especially veins in vivo, may depend on their location and orientation. Many arteries, veins and nerves are located in close proximity to each other anatomically and the effects of such positioning cannot be analyzed thoroughly by in vitro studies. This close proximity between arteries and veins in vivo suggests that there may be a tethering of veins to arteries that is mediated by the surrounding extracellular matrix (ECM). This tethering may be important in regulation of mechanotransduction by smooth muscle and endothelial cells altering vessel wall metabolism.

Interpretation of the relationship between internal and external mechanical loading on vessel wall structure and properties is complicated by the lack of calibrated methods to measure the mechanical properties of vessels in vivo. In this paper we extend earlier findings on the use of vibrational optical coherence tomography (VOCT) to determine the mechanical properties of vessel walls in vivo [13]. We compare the behavior of arteries and veins that are in close proximity to each other in an attempt to understand how the surrounding extracellular matrix may influence vessel wall mechanical properties and mechanochemical transduction that occurs throughout mammalian vascular system.

Subjects

In vivo analysis of radial, brachial, femoral, and posterior tibial arteries and veins was conducted on 3 subjects (2 men and 1 woman) after informed consent was obtained. Arterial pulse was palpated to determine the location of the arteries. The precise location of arteries and veins was further identified using ultrasound and a linear 7.5 MHz probe. An ultrasound image was obtained at the site where the vibrational measurements were made.

Measurement of Resonant Frequency and Elastic Modulus

VOCT is a non-invasive and non-destructive method that uses audible sound from a speaker to create a transverse tissue displacement as described previously [13-16]. The displacement is detected using reflected infrared light that is analyzed by optical coherence tomography. The displacement peaks as a function of frequency create a mechanical spectrum that defines the characteristics of each major structural component of tissues. The resonant frequency of a component is defined as the frequency at which the maximum displacement is observed. The measured resonant frequencies of tissue components are converted into modulus values using a calibration equation for soft tissues. Calibration is based on in vitro uniaxial mechanical tensile testing and VOCT measurements made on the same material [13-16]. This data is used to develop equation (1) for soft tissues. Since most soft tissues have a density very close to 1.0, equation (1) is valid for the majority of tissues found in the body; where the thickness d in meters is determined from OCT images, fn² is the square of the resonant frequency, and E is the elastic modulus in MPa.

Soft Tissues E*d=0.0651*(fn²) + 233.16 (1)

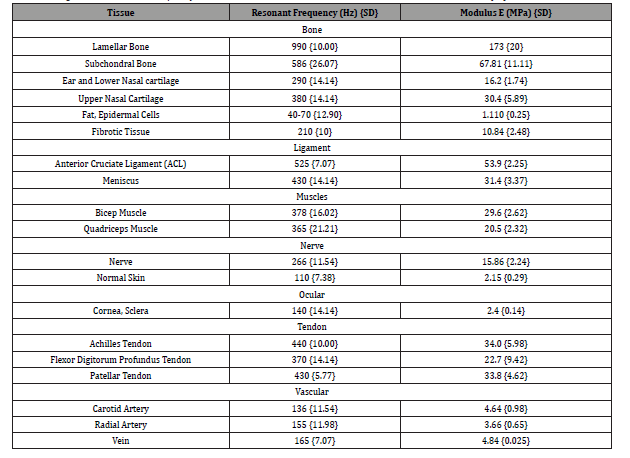
The resonant frequency of each component of the sample is determined by measuring the displacement of the tissue resulting from sinusoidal driving frequencies ranging from 30 Hz to 20,000 Hz, in steps of 10 to 100 Hz. The maximum displacement occurs at the resonant frequency, fn, [13-16]. The resonant frequencies and moduli of human tissues previously measured using VOCT are listed in (Table 1) [13].

Table 1: Assignment of Resonant Frequency Peaks and Associated Moduli Based on Vibrational OCT Measurements [13].

Measurement of Loss Modulus as a Fraction of the Elastic Modulus

Viscous loss measurements, which are related to the energy dissipation of a material, are reported as a fraction of the elastic modulus. Samples are subjected to three pulses of audible sound waves at frequencies between 50 and 500Hz in steps of 20 Hz. The viscous component of the viscoelastic behavior is obtained from the driving frequency peak by dividing the change in frequency at the half height of the peak (i.e. 3db down from maximum peak in power spectrum) by the driving frequency after the third pulse has ended. This method is known as the half-height bandwidth method discussed by Paul Macioce [13].

In vivo elastic and viscous properties of arteries and veins were measured using VOCT and a Vibrational Otoscope (OptoVibronex, LLC.) An oscillating sound wave is applied to the skin and the reflected wave is analyzed based on the vibrations measured at the skin surface. The frequency at which the maximum displacement is observed for each tissue component is specific and termed the resonant frequency.

Figure 1 shows a weighted displacement versus frequency plot for normal skin over the radial artery in the hand. Note the peaks at about 70 Hz (cells), 100 Hz (dermal collagen) and 150Hz (blood vessels) were assigned based on previous calibration studies [13]. Figure 2 shows an image of the femoral artery and vein and the dimensions from one of the subjects. Note the close proximity of both the vessels in vivo with a distance of about 9.5mm between the centers of these vessels; the artery and vein appear to be connected on the outer surface by extracellular matrix (ECM). Figure 3 shows a plot of weighted displacement versus frequency for the femoral artery at the rest and after running for 5 minutes with the subject’s heart rate increasing to about 100. In Figure 3 at rest, peaks are seen at 70 (cells), 110 (dermal collagen) and 160 (blood vessel). Between 40 and 70 Hz the resonant frequency corresponds to cells, 100 to 120Hz to dermal collagen, 140 to 160Hz to blood vessels, and 250-270Hz to nerves [13]. After exercise, peaks are seen at 70 (cells), 100 (dermal collagen) and 180 (blood vessels) Hz. Note the increase in the vascular peak frequency from 160 Hz to 180 Hz which is consistent with increased arterial stiffness with increased blood flow. The data in Table 2 indicates that an increase in the blood pressure due to running increases the vascular stiffness by about 2 MPa or about 40% for a number of different arteries.

Table 2: Modulus values calculated from equation 1 for in vivo measurements on arteries and veins before and after exercise using vibrational optical coherence tomography.

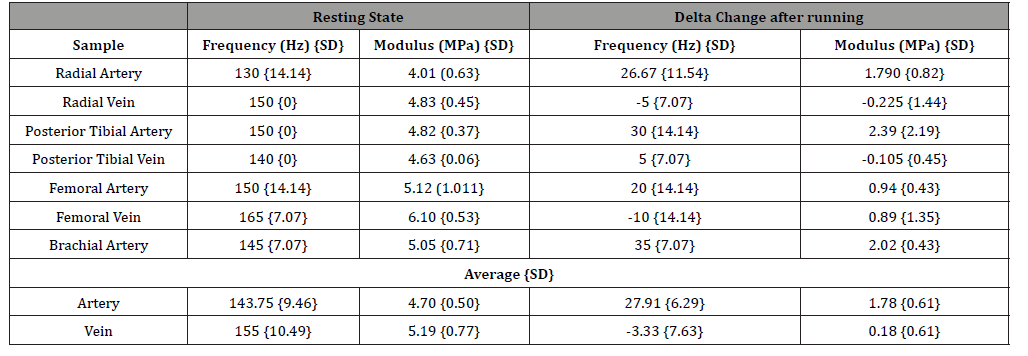
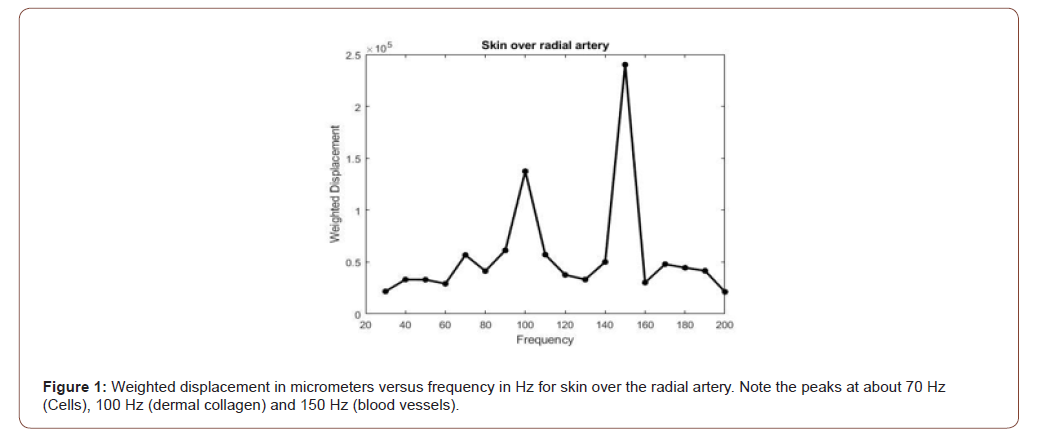
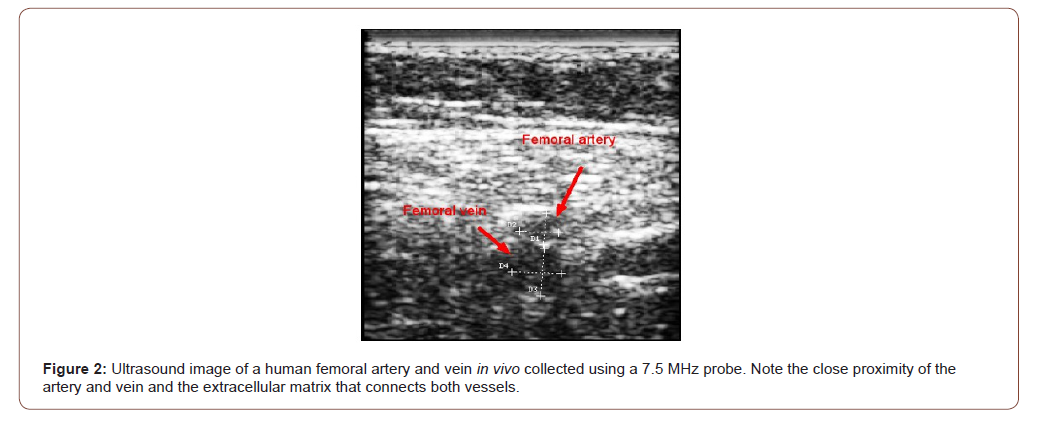
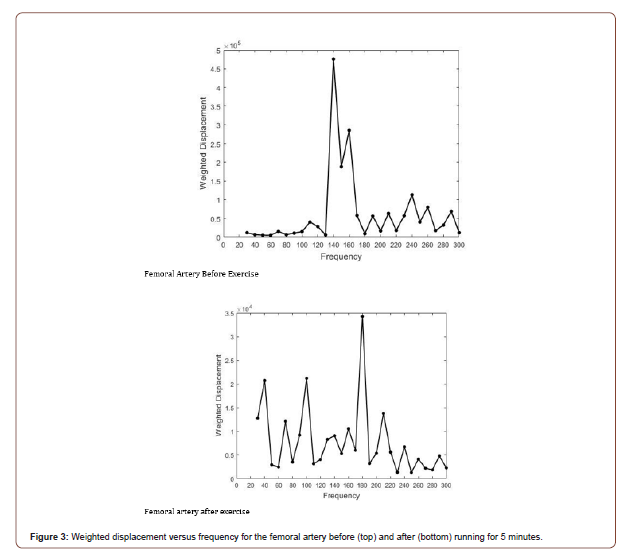
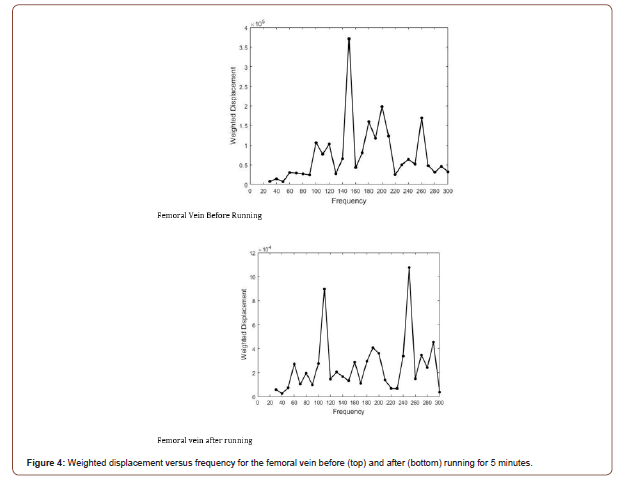
Figure 4 shows the behavior of the femoral vein in vivo before and after running for 5 minutes. The vascular peak is 150 Hz before running and about 160 Hz after running. Note that in spite of a higher heart rate the stiffness of the venous peak does not appear to change significantly as shown by the data in Table 2. The stiffness of all the veins studied did not change after running as demonstrated by the data in Table 2. This observation suggests that a higher venous blood volume does not produce a significant change in the stiffness of the vein only a change in shape. Arteries and veins both in the legs and arms evaluated in this study have similar mechanical properties in vivo as indicated by the data in Table 2 for the radial, brachial, femoral, and posterior tibial artery and veins. The average value of the elastic modulus observed for arteries was 4.70 +/- 0.5 MPa and for veins 5.19 +/- 0.77 MPa. This similarity between the modulus at rest of arteries and veins indicates that ECM tethering may provide a means for eliminating stress concentrations that may occur in anatomic areas where arteries and veins are in close proximity.

Figure 5 shows a plot of loss modulus as a fraction of the elastic modulus as a function of frequency before and after running for the femoral artery of a subject. Note most of the energy loss occurs at low frequency and appears similar to the energy dissipated by skin at low frequencies [13-16]. The plots for femoral vein before and after running are similar to Figure 5. This data suggests that most of the energy dissipation by blood vessels in normal subjects occurs via the ECM that ties them together. In skin, this dissipation has been attributed to fluid flow and proteoglycan rearrangement on the surface of collagen fibrils [13-16].

The role of elastic tissue, collagen, and smooth muscle in the mechanical properties of vessel walls are important parameters needed to evaluate the state of health and disease of human blood vessels [9, 10, 17]. The mechanical properties of relaxed vessels have been attributed to the properties of elastic tissue and collagen [18]. Elastic tissue is believed to bear the load at low distention pressures [19] and has a modulus of 0.5 MPa [18]. In contrast, at high extensions the behavior of vessel walls has been attributed to collagen [19, 20] and a collagen and smooth muscle unit containing series elements [9, 10]. The modulus of collagen fibers in skin, cornea and sclera is estimated to be about 2 to 3 MPa under physiologic conditions [13-16]. It can be as high as about 10 MPa in scar tissue or 34 MPa in tendons and ligaments under physiological conditions [13-16]. The relationship between collagen network structure and mechanical properties is an important parameter to understand elastic energy storage and auxiliary pumping efficiency of elastic arteries.

The results reported in this paper suggest that both arteries and veins in vivo exhibit elastic moduli that are between 4 and 5 MPa which is significantly higher than the low strain modulus observed in the stress-strain curves of human or pig vessels studied in vitro [10, 11]. This suggests that in pulsating arteries and in veins the vessel wall is under tension due to the active contraction of smooth muscle cells. Tethering between arteries and veins via the surrounding ECM produces no stress concentrations at the interfaces between these vessels. Based on ultrasound images, arteries and veins appear to be connected to each other via the surrounding ECM. Under these conditions the smooth muscle must contribute significantly to the behavior of both arteries and veins since these vessels are anatomically in parallel with each other. Thus, stretching of arteries transmits stress to the veins that are in close proximity via the ECM that tethers them together. Arterial diameter increases that result from increased blood flow lead to increases in moduli. Increased venous blood flow causes changes in the shape of veins without an associated increase in the modulus.

The modulus of collagen that is found in other tissues (dermis and cornea) is much less than the modulus of blood vessels reported in this paper (4 to 5 MPa) [13]. This suggests that the ECM found between connecting the arteries and veins not only provides a tethering effect that transmits forces between arteries and neighboring veins. It also links them in a parallel arrangement and acts to transmit mechanical loading throughout the vasculature, up- and downstream. Arterial diameter increases store energy elastically while tethering by the surrounding ECM would limit tissue deformation and transmit loading forces to other parts of nearby arteries and veins. This would occur when the mechanical pulse wave moves up and down the vessel wall providing loading to segments of the wall not directly linked anatomically. In addition, diameter increases and increases in the arterial moduli would change stresses in the tethered veins and in other segments of the artery that would alter mechanotransduction pathways. This would up- or down-regulate tissue metabolism that could lead to either new vessel formation or to stenosis and calcification under abnormal flow conditions. Increased blood volume and arterial diameter increases could stimulate new capillary and venule formation that would be needed for sustained oxygen delivery to tissues during prolonged exercise.

The mechanical coupling between the cellular components and collagen and elastic fibers in the ECM has been addressed in several studies. A number of in vitro studies using arterial strips have been performed to assess the relative contribution of each of these components [6, 20-24]. An elastic component has been proposed to be connected in series with smooth muscle or with a parallel elastic element and smooth muscle [21]. A possible basis for the elastic component comes from structural studies that suggest that aortic smooth muscle cells are bound together in series by their basement membranes and collagen fibrils [22]. Based on these anatomical studies, the vascular peak observed in this study between 140 to 160 Hz probably represents the series elastic component of collagen and smooth muscle with a modulus of 4 to 5 MPa.

Isometric smooth muscle contraction has been shown in vitro to increase the arterial wall modulus from 3.84 to 9.92 MPa [22]. The large increases in stress and modulus that occur with isometric smooth muscle contraction are due entirely to the generation of tension in the smooth muscle and its associated series elastic component [20]. Activation of smooth muscle increases the elastic modulus from a maximum of about 4 MPa for the whole canine carotid artery to almost 13 MPa in vitro [6]. Our results suggest that the collagen and smooth muscle cell networks are in series in the vessel wall model. Since veins have lower smooth muscle content [20], they do not get appreciably stiffer with exercise.

The relationship between internal and external mechanical loading on vessel wall mechanical properties has been studied in vivo using VOCT. In this paper we extend earlier findings of the mechanical properties of vessel walls in vivo [13]. Our results suggest that many arteries and veins are tethered together via the surrounding ECM, both laterally and longitudinally. The mechanical properties of this lateral tethering are influenced by the series connections between collagen fibers of arterial walls and smooth muscle cells that give rise to a mechano-vibrational peak at 140-160 Hz. This peak has a modulus of between 4 and 5 MPa. Exercise increases the arterial modulus by about 2 MPa and this change in stiffness can influence the surrounding arteries and veins via the tethering by the ECM. Lateral tethering results in effective stress transfer between arteries and neighboring veins limiting stress concentrations and transmitting mechanical information between the arterial and venous sides of the vascular system. Longitudinal tethering transmits loading information up and down the arterial system via wall shear wave propagation. This wave propagates up and down the vascular tree. In this manner external and internal mechanical information can influence anabolic and catabolic changes via mechanotransduction. These loading changes lead to changes in the composition and mechanical properties of the overall vascular system.

None.

No conflict of interest.

1. Patel DJ, Janicki JS, Carew TE (1969) Static anisotropic elastic properties of the aorta in living dogs. Circ Res 25: 765-775.
2. Kalath, S, Tsipouras P, Silver FH (1986) Non-invasive assessment of aortic mechanical properties. Ann Biomed Eng 14: 513-524.
3. Langille BL (1996) Arterial remodeling: relation to hemodynamics. Can J Physiol Pharmacol 74: 834-841.
4. Lehoux S, Esposito B, Merval R, Loufrani L, Tedgui A (2000) Pulsatile stretch-induced extracellular signal regulated kinase 1/2 activation in organ culture of rabbit aorta involves reactive oxygen species. Arterioscler Thromb Vasc Biol 20: 2366-2372.
5. Hipper A, Isenberg G (2003) Cyclic mechanical strain decreases the DNA synthesis of vascular smooth muscle cells. Pfluegers Arch 440: 19-27.
6. Dobrin PB, Rovick AA (1969) Influence of vascular smooth muscle on contractile mechanics and elasticity of arteries. Am J Physiol 217: 1644-1652.
7. Silver FH, Christiansen DL, Buntin CM (1989) Mechanical properties of the aorta: A review. Crit Rev Biomed Eng 17: 323-358.
8. Silver FH, Snowhill PB, Foran DJ (2003) Mechanical behavior of vessel wals: A comparative study of aorta, vena cava, and carotid artery. Annals Biomed Eng 31: 793-803.
9. Silver FH, Siperko LM (2003) Mechanosensing and Mechanochemical Transduction. Crit Rev Biomed Eng 31: 255-331.
10. Snowhill PB, Foran DJ, Silver FH (2004) A mechanical model of porcine vascular tissues-part I: determination of macromolecular component arrangement and volume fractions. Cardiovascular Engineering 4(4): 281-294.
11. Snowhill PB, Silver FH (2005) A Mechanical Model of Porcine Vascular Tissues-Part II: Stress–Strain and Mechanical Properties of Juvenile Porcine Blood Vessels. Cardiovasc Eng 5: 157-169.
12. Lang RM, Cholley BP, Korcarz C, Marcus RH, Shroff SG (1994) Measurement of Regional Elastic Properties of the Human Aorta A New Application of Transesophageal Echocardiography With Automated Border Detection and Calibrated Subclavian Pulse Tracings. Circulation 90(4): 1875-1882.
13. Silver FH, Horvath I, Kelkar N, Deshmukh T, Shah R (2020) In Vivo Biomechanical Analysis of Human Tendon Using Vibrational Optical Coherence Tomography: Preliminary Results, Tomography: Preliminary Results. J Clin Cases Rep 4(1): 12-19.
14. Silver FH, Shah RG, Benedetto D (2018) Non-Invasive and Non-Destructive Determination of Corneal and Scleral Biomechanics Using Vibrational Optical Coherence Tomography: Preliminary Observations. Mater Sci App 9(7): 657-669.
15. Silver FH, Shah RG, Richard M, Benedetto D (2019) Comparison of the virtual biopsies of a nodular basal cell carcinoma and an actinic keratosis: Morphological, cellular and collagen analyses. Adv Tissue Eng and Regen Med 5: 61-66.
16. Silver FH, Shah RG, Richard M, Benedetto D (2019) Comparative “virtual biopsies” of normal skin and skin lesions using vibrational optical coherence tomography. Skin Res Tech 25: 743-749.
17. Buntin CM, Silver FH (1990) Non-Invasive Assessment of Mechanical Properties of Peripheral Arteries. Annals Biomedical Engineering 18: 549-566.
18. Dunn MD, Silver FH (1983) Viscoelastic Behavior of Human Connective Tissues: Relative Contribution of Viscous and Elastic Components. Connective Tissue Research 12: 59-70.
19. Roach MR, Burton AC (1957) The reason for the shape of the distensibility curves of arteries. Can J Biochem Physiol 35: 681-690.
20. Silver FH, Kato YP, M Ohno, Wasserman AJ (1992) Analysis of mammalian connective tissue: Relationship between hierarchical structures and mechanical properties. J Long Term Eff Med Implants 2: 165-198.
21. Dobrin PB (1978) Mechanical properties of arteries. Physiol Rev 58(2): 397-460.
22. JM Clark, S Glagov (1979) Structural integration of the arterial wall. I. Relationships and attachments of medial smooth muscle cells in normally distended and hyperdistended aortas. Lab Invest 40: 587-602.
23. Clark JM, S Glagov (1985) Transmural organization of arterial media: The lamellar unit revisited. Arteriosclerosis 5(1): 19-34.
24. Bank AJ, H Wang, JE Holte, K Mullen, R Shammas, et al. (1996) Contribution of collagen, elastin, and smooth muscle to in vivo human brachial artery wall stress and modulus. Circulation 94: 3263-3270.