Case Study of An Interstellar Flight: Unmanned Interstellar Mission to the Star System Lalande 21185

“Le Reve D’etoiles” or the “Dream of Stars” has been a major dynamic force, which has propelled mankind forward since the dawn of civilization. Since the inception of the first known structured settlement at Gobeklitepe, it can be seen from the archaeological digs that several paintings and inscriptions exist to show that mankind has always looked upon the stars and sometimes even worshipped them. Even with ancient civilizations such as Babylonians, Assyrians, Sumerians, Mayans, and Egyptians; the stars always represented a means of reaching far into the heavens as the twinkling stars represented heavenly light and as a result serious astronomical work was conducted in order to understand the meaning behind the stars [1]. In fact, it must be stated that Sumerians have executed a number of interesting findings related to the cosmos and physics, and some of their findings even constitute a very primal form of the String Theory that we use today to try to explain the universe.

Today, we know for a fact that billions of stars or more exist in our galaxy alone and it is hard to calculate the total number of stars, which may be present in the universe. Especially, with the advancements after the second half of the 20th century, it has become a realistic dream of mankind to visit those stars someday. While the idea of visiting stars has become a very common concept within the purview of science fiction; real science is quite far behind in achieving these objectives. Within today’s standards, even achieving a manned flight to any destination beyond the destination of Moon is a very important challenge, since it requires quite advanced mission planning that incorporates things such as protection from ra- diation exposure on prolonged missions, life support requirements such as food, water, and exercise for multiyear missions, as well as sufficient power sources with backups to provide power for the internal and external systems of the spacecraft.

Naturally, the objective is to make sure that the mission can be concluded within an acceptable time frame, as every extra day that is added to the mission stage will cause severe problems to the operability of the spacecraft and too long a period can easily cause the mission to fail completely. Thus, from the above requirements, it can become almost impossible to mount a mission that can require several years to complete. However, if the interstellar mission is to be idealized, then the most important component is the specific impulse, which will allow for mission time to be shortened as much as possible. Of course, it is also important that the acceleration and deceleration components are calculated in a way to suit the requirements for such a high-speed mission [2, 3].

Why is Interstellar Travel Important for Mankind?

Interstellar travel, the ability to travel between star systems, holds tremendous importance for humanity’s future. Here are some key reasons why interstellar travel is significant:

a. Expansion and Colonization: Interstellar travel opens up the possibility of exploring and colonizing other star systems. As Earth’s population continues to grow, the availability of resources and living space becomes a pressing concern. Interstellar travel could provide new habitable worlds or resources that could sustain human life, offering a potential solution to overpopulation and resource depletion.

b. Scientific Exploration: Interstellar travel would allow us to explore and study exoplanets, which are planets outside our solar system. By visiting these distant worlds, we can gain insights into their composition, atmosphere, potential for harboring life, and a broader understanding of the diversity of planetary systems. This knowledge can deepen our understanding of the universe and our place within it.

c. Search for Extra-terrestrial Life: Interstellar travel would greatly enhance our ability to search for extraterrestrial life. By visiting exoplanets or their moons, we can directly study the conditions and environments that may support life. The discovery of life beyond Earth would have profound implications for our understanding of biology, evolution, and the prevalence of life in the universe.

d. Technological Advancements: The pursuit of interstellar travel drives the development of advanced propulsion systems, spacecraft design, life support systems, and other technologies that would have far-reaching applications on Earth. The challenges of interstellar travel push the boundaries of scientific and engineering disciplines, fostering innovation and leading to technological breakthroughs that could benefit various industries and improve the quality of life for humanity.

e. Survival and Long-term Sustainability: Interstellar travel holds the potential for ensuring the long-term survival of humanity. By establishing colonies on other habitable worlds, we could mitigate the risks associated with natural disasters, cosmic events, or the potential decline of Earth’s habitability. Interstellar travel would provide a means to safeguard the human species and preserve our knowledge and culture for future generations.

f. Philosophical and Existential Significance: Interstellar travel raises profound philosophical questions about our place in the universe, the nature of life, and our cosmic significance. Exploring other star systems would challenge our perspectives, broaden our horizons, and deepen our understanding of the fundamental questions about our existence.

While interstellar travel poses significant technological and logistical challenges, its pursuit pushes the boundaries of human potential and offers a future where humanity could explore and thrive beyond the confines of our solar system.

The importance of Lalande 21185 as a destination for an interstellar mission

The star Lalande 21185 is important for mankind due to its status as one of the nearest known stars to our solar system. Here are some reasons why it holds significance:

a. Proximity: Lalande 21185 is located relatively close to our solar system, approximately 8.31 light-years away. In astronomical terms, this is considered a short distance. Proximity is crucial for future space exploration and potential interstellar travel. It offers a reachable target for studying exoplanets, conducting research, and potentially sending missions to explore this stellar system.

b. Exoplanet Exploration: Lalande 21185 presents an opportunity to search for exoplanets within its planetary system. Exoplanets are planets that orbit stars outside our solar system. Studying exoplanets helps us understand the diversity of planetary systems, the potential for habitability, and the prevalence of life in the universe. Proximity to Lalande 21185 enables scientists to observe its exoplanets more directly, potentially gathering valuable data about their atmospheres, compositions, and potential for supporting life.

c. Technological Development: The study of Lalande 21185 and its planetary system stimulates technological advancements. Exploring distant stars and exoplanets requires advanced telescopes, instruments, and spacecraft. The pursuit of knowledge about Lalande 21185 pushes the boundaries of astrophysics, astronomy, and space exploration technologies, which can have broader applications and benefits for humanity.

d. Insights into Stellar Evolution: Close examination of Lalande 21185 provides insights into stellar evolution. By studying these types of stars, scientists can gain a better understanding of the life cycles of stars, including their formation, evolution, and eventual fate. This knowledge contributes to our understanding of the universe, star formation processes, and the factors that influence the habitability of exoplanets.

e. Human Space Exploration: Lalande 21185’s proximity could be relevant to future human space exploration. Although the star system is still quite distant, its relative closeness compared to many other stars makes it a potential target for future manned missions. Exploring Lalande 21185 and its potential exoplanets could be an important step toward expanding our presence beyond our solar system.

f. Ability to Expand Beyond to our Galaxy: Lalande 21185 is near enough to our solar system to be within the 10 light years proximity of influence, yet it is far enough to become a foothold to outer regions of our local interstellar space giving mankind an ability to not only study the Lalande 21185 system, but also to be able to scan for other stars and exoplanets and even for presence of other civilizations beyond our local sphere of influence.

While Lalande 21185 is just one star among the vast number of celestial objects in the universe, its proximity makes it an enticing target for scientific exploration and a potential stepping stone for future space endeavors. Its study could significantly contribute to our knowledge of exoplanets, stellar evolution, and the prospects of interstellar travel.

Location of Lalande 21185

The objective of this paper is to focus on a mission to Lalande 21185, which is approximately 8.29 light-years from Earth and it is the fourth closest star system to Earth and our solar system in general. It is located in the Ursa Major constellation. The star Lalande 21185 was first discovered and cataloged by Joseph-Jérome Lefran ais de Lalande (1732-1807), who became director of the Paris Observatory in 1795. There is a lot of data, which has been accumulated through astronomical observations regarding Lalande 21185, which has been observed to be a red dwarf star [4]. Some of the interesting properties of Lalande 21185 can be seen below including the star as well as the system. Lalande 21185 is also known as NSV 18593, GC15182, and Gliese 411. The coordinates for Lalande 21185 are (11:03:20.19+35:58:11.55, ICRS 2000.0).

Table 1: Stellar Data for Lalande 21185 and its System.

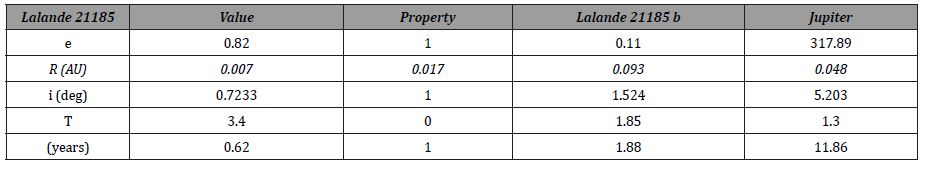
Properties of Lalande 21185

While Lalande 21185 is the fourth closest star to our solar system, it has many interesting properties which make it an interesting destination for astronomers, astrophysicists as well as Astronautical engineers. Many people concentrate on more closer destinations such as the Alpha Centauri system as well as Barnard’s Star, but actually, in many aspects, Lalande 21185 is more interesting.

The surface temperature of Lalande 21185 is around 3,383 K and it is classified as a Red Dwarf star. Lalande 21185 has a mass that is equivalent to the 46% of the sun and as a result, it can be categorized as one of the bigger Red Dwarfs found in our galaxy [5].

Lalande 21185 usually emits its radiation in the form of infrared, but a very small portion of it is also emitted in the visible light spectrum. It is considered to be approximately 200 times dimmer than our sun, nevertheless, it is still one of the brighter Red Dwarf stars found in our Milky Way Galaxy.

It is also classified as a Thick Disk star and as a result, its orbit has a very high eccentricity as to the galactic plane. This makes it interesting since it is considered as an older star with a quaint motion. Actually, the orbital path of Lalande 21185 is made in such a way that it is always coming closer to our sun. In fact, our sun and Lalande 21185 are coming closer to each other at a speed of 85 kilometers per second [5]. Hence, it is expected that by the year 22,000 AD, the Lalande 21185-star system may be as close as Alpha Centauri, which is at 4.5 light years away from us currently.

In general, Lalande 21185 is almost twice as old as our sun. From a metallic composition point of view, Lalande 21185 is metal- poor as it has 63% of the metallicity of the sun. This can be considered to be expected due to its age, since most of the materials would have been burned up in the fusion reaction that has continued for eons. Now as a Red Dwarf star, it has a limited composition [5]. However, the study of Lalande 21185 can be crucial in understanding the formation of the universe and the life cycle of stars, and this would make such an interstellar mission to become viable as it would expand our scientific horizons.

Planetary system of Lalande 21185

One of the things that is found to be interesting about Lalande 21185 is the fact that it is purported to have a planetary system. The claim was first put forward by the Dutch astronomer Peter van de Kamp in 1951, by observing the wobbling motion of the star’s movement, which usually indicates a planetary companion. Kamp’s observations stated that there was a possibility of two planets orbiting Lalande 21185.

George Gatewood also made similar observations by stating that as many as three planets may be orbiting Lalande 21185 and it caused quite a stir and academic excitement in the academic community during 1996. The latest discovery came in 2002 by the Italian astronomer Christiano Cosmovici as he found traces of water vapor in the Lalande system, which could be indicative of a planet as well as indicative of a cometary collision in the system. Right now, mostly the assumption that two planets orbit around Lalande is the accepted norm in the astronomy community [5].

The first planet Lalande 21185a is around 2.2 AU of distance from the star. Due to the high distance, as well as the state of the red dwarf having a lesser temperature, causes this planet to be extremely cold at this distance. Lalande 21185a has around 90% of the mass of the planet Jupiter and the temperature at the surface of the planet is estimated to be less than 1500 Centigrade. It has a highly circular orbit, which allows it to circle its star in roughly 5.8 years.

The second planet around Lalande 21185 is called Lalande 21185b and it is situated at a 10 AU distance from Lalande 21185. Lalande 21185b has around 160% of the mass of Jupiter as determined by the tug that it creates in the system, which makes it a giant planet. Lalande 21185b takes around 30 years to complete one orbit around Lalande 21185 and it is a very cold planet due to the high distance from its parent star. Its surface temperature is speculated to be around 370 K. Both of these planets around Lalande 21185 have been found by using astrometric methods. They have been verified several times with other observations and studies. While there is speculation that a third exoplanet may also be found in the system, until an interstellar mission reaches there, it will be hard to determine exactly. However, all of the information about Lalande 21185 points out that it is a fascinating star system worth discovering.

Possibility of life in the Lalande 21185 system

Under these conditions, it is quite hard to state that any signs of life can be found in the Lalande 21185 system. The star itself is a red dwarf star which reduces the availability of necessary electromagnetic radiation that acts as an energy source for life. In fact, even the visible light spectrum is almost nonexistent and the state of the star causes very low temperatures at its planets, which can be considered as gas giants [5].

Hence, it can be safely said that the Lalande 21185-star system will not harbor any form of conventional life. However, there is still some speculation stating that Lalande 21185b might have some other forms of life, which may have been created by the tidal forces that may be prevalent in the system. Naturally, more data is needed to make a more concrete determination of the fact.

Specific impulse

When talking about interstellar travel possibilities, then it is essential to speak about special propulsion techniques that transcend far beyond chemical propulsion. As it is known from the fundamentals of rocket propulsion, very high specific impulses as well as high acceleration levels must be required, so that proper results can be achieved.

The specific impulse of a spacecraft depends directly on the energy density (J) of the flow that is being utilized. Hence, the equation of the specific impulse can be written as [6]:

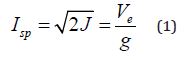
Where J is the Energy Potential and Ve is the exhaust velocity of the rocket

Any specific impulse below 10,000 would not even be discussable, as an interstellar trip would take a very long time, which could not be manageable with a low specific impulse by any standards concerning life support, as well as the logistics of the spacecraft.

Besides the specific impulse, which is a passive indication of a speed of a spacecraft, it is also essential to think about the acceleration of a spacecraft. In conventional spacecraft dynamics, initial momentum is given and small adjustments are made and the spacecraft continues on its path unimpeded until it reaches the gravitational influence of a body.

Hence, the initial speed imparted by the thrusters is important for creating a high specific impulse. However, since the distances in interstellar travel involve light years, which is time that needs to pass while traveling at light speed, more exotic means are needed. Instead of using an initial thrust and relying on it to produce high specific impulses, constant thrust can be applied to the spacecraft to impart acceleration.

For example, if you apply constant thrust at ½g; then naturally the spacecraft will keep gaining velocity since there are no unopposing forces. Thus, after months of acceleration, then the spacecraft would be gaining such high speeds and a high specific impulse, it can be possible for it to continue on an interstellar journey for long periods of time [7].

Hence, the question becomes of applying constant thrust nonstop for long periods of time to reach semi-relativistic speeds (1% to 40%) so that travel time would become meaningful. Of course, it would still involve decades of travel, but even with today’s technology, it can become manageable as long as the necessary budget requirements are met [7, 8].

Naturally, special thrusters are needed that use less amount of fuel and supply large amounts of energy to the spacecraft. The engine system must be able to keep up with constant acceleration and it must also be able to provide enough power to supply the requirements of life support systems, navigational systems, onboard computers, onboard communication systems, as well as payload systems. Thus, using a method that has a chance of supplying such power will be necessary for the operational efficiency of the spacecraft. It will require some special technology, but it is certainly manageable within today’s technology and budget [9].

Relativistic effects during interstellar travel

If such high velocities and acceleration levels are to be discussed, then the calculations for such an interstellar trip will need to be done with the help of Einstein’s Theory of Relativity. As such high speeds are reached, the theory of relativity takes effect and as a result, there are certain properties which are changed. Some changes that take place at near relativistic speeds include dilation of time inside the spacecraft for the crew as well as expansion of mass for the spacecraft. While at the speeds discussed above, the mass expansion would be negligible, while the time dilation would not be.

For the astronauts onboard the spacecraft, the time will pass differently. Due to the Theory of Relativity, it would seem as the time would pass slower as compared to the time passing on Earth for the mission control crews. In relativistic physics, this has already been discussed as the twin paradox. In the twin paradox, one twin remains on Earth, while the other twin travels at relativistic speeds. When the space-traveling twin returns, he sees that his twin who remained on Earth has aged decades, while only a few years have passed for the twin in space.

The same phenomena would be applicable to astronauts who are going on interstellar travel to a destination at semi-relativistic speeds.

In a way, this is advantageous as the time passes for the astronauts can be reduced, and the corresponding power and life support requirements can be reduced as well. This is an important advantage, as it allows for more efficient planning of a mission with lesser resources. As a result, it can be stated that the time dilation effects would have to be counted as even a reduction of one year in the ship’s time could mean so much in terms of reducing the needs in terms of logistics (such as food, air, heating, etc.) onboard the spacecraft.

However, it also needs to be understood that the time on Earth in mission Control would pass normally. Nothing can be done to mitigate this as this will have to be taken as an integral part of the mission. Hence, from the point of view of data retrieval, the mission control would have to wait a significant amount of time before they will be able to receive any meaningful data from the star systems.

Thus, when planning for an interstellar mission, several parameters need to be known beforehand such as the maximum attainable acceleration, the maximum attainable speed, the maximum percentage of relativistic speeds, etc. Of course, while doing the calculations, it is essential to also think about acceleration as well as deceleration, since the spacecraft cannot be stopped suddenly. As a result, it is essential to make sure that acceleration to the halfway mark is taken and deceleration is taken from that point or a more aggressive deceleration policy can be adapted as well to conserve time.

Since the approachable speeds are in the range of 0.1c to 0.4c in most advanced propulsion methods, it is essential to incorporate the effects of relativity into the equations. Naturally, the most important relativistic effects are relativistic mass expansion as well as relativistic time dilation. Both of these effects will have a direct effect on the interstellar mission. The relativistic mass expansion will indirectly cause more kinetic energy to be used in order to accelerate the spacecraft. Hence, in concordance with this, it may be necessary to increase the thermal capacity of the nuclear reactor in order to pump more kinetic energy into the flow.

The mass expansion and velocity changes at relativistic speeds are given by [10]:

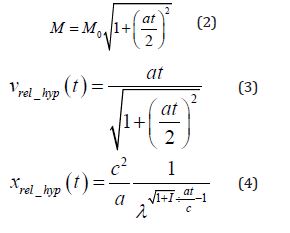
Where

M0= Mass of the spacecraft

M= Mass Expansion

a= Acceleration of the spacecraft

t= Time Expansion

c= Speed of Light in vacuum

Another point of interest is the relativistic time dilation. In a way, this will be an important advantage to the interstellar mission. By effectively increasing the time dilation, the amount of time the crew feels onboard the spacecraft will be decreased as compared to Earth-bound time. This will actually reduce the physiological effects as well as the psychological effects of long, space-bound travel on the crew of the spacecraft. The equation for shipboard time is given by the following equation:

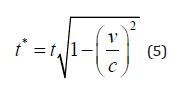
Where:

t* = Ship time i.e. the time with respect to the crew members in the spacecraft

t = Earth time.

Hence, both the effects of the time dilation as well as the mass expansion will need to be incorporated into the design of the mission parameters for the best results.

Nuclear propulsion in interstellar travel

While many exotic forms of interstellar propulsion exist on the drawing boards, the purpose of this paper is to discuss a feasible scenario of reaching Lalande 21185 by using propulsion technologies that are within the reach of 21st century. In order to achieve this, existing forms of propulsion are discussed and more exotic means of propulsion such as Alcubierre’s warp drive will be discounted for now.

One such method that is available within the grasp of today’s technology is nuclear propulsion techniques. This is essential in one aspect that nuclear propulsion techniques have been experimented with and analyzed since the 1960s and a large amount of data has been collected in this regard.

We already know that any proportion methods that use inertia must be able to expel any propellant from the spacecraft as fast as possible. As it can be ascertained, in order to achieve this, it is essential to heat up the propellant to very high temperatures. As the gas molecules heat up, they will turn this heat energy into kinetic energy and they will turn into this frenzied state where they will vibrate at high speeds. If they are discharged at those high speeds, naturally the spacecraft will be given a significant push forward and as a result, the spacecraft will gain velocity. As the temperatures in the propellant increase, this will cause a direct increase in the speed of the exhaust velocity itself, which in turn will increase the specific impulse of the spacecraft. Thus, nuclear methods have the best chance of heating the propellant to very high temperatures, so that extremely high speeds can be reached [11].

Naturally, the first nuclear propulsion method that comes to mind is the fusion drive. With Fusion, it is possible to reach very high temperatures that can give a significant boost to the exhaust velocity of the spacecraft. However, these temperatures are unmanageable with today’s technology. While it is possible to use special magnetic fields to contain the fusion reaction itself, the temperatures would turn the outer casing to plasma instantaneously. Hence, a lot of time needs to be spent on containing the fusion reaction itself, so that appropriate results can be obtained.

Fission propulsion techniques are the second techniques that come to mind. However, conventional fission techniques are also insufficient to part enough energy to the propellant as the speeds reached won’t be that high for interstellar purposes. But as an alternative, it is possible to use advanced fission propulsion techniques such as gaseous core propulsion using Uranium Hexafluoride to achieve temperatures in the order of 10,000 degrees Kelvin. This is a temperature, which is manageable with today’s technology and as a result, long-duration interstellar travel can be planned with this technology with some improvements.

Gaseous core nuclear propulsion

It is important to understand that gaseous core technology is a means to interstellar travel, but it will need some adjustments from present-day technology to achieve the desired purpose. First of all, the gaseous core fission uses gaseous fission fuels instead of solid fission fuels. This gives a very powerful solution, since solid nuclear fuel rods would melt after a couple of thousand degrees of Kelvin. However, with gaseous fuels, the fission reaction would take place with gaseous nuclear fuel such as Uranium Hexafluoride or Uranium Tetrafluoride [11].

The utilization of Uranium Hexafluoride is a great advantage as the temperature limitations that are found in regular space reactor applications are easily overcome and as a result, higher specific impulses can be obtained. Moreover, it can be possible to have a long period of acceleration, since the fission of Uranium Hexafluoride would give sustained thrust to the spacecraft.

Moreover, the fission cross sections of gaseous core nuclear fission reaction are also high as compared to classic fission, and as a result, the fission reaction will have a higher reactivity constant and the reactor can be operated at a slightly more supercritical condition as compared to regular fission reactor. A supercritical condition in a nuclear reaction depicts a higher level of fission reaction that outputs more power as compared to a critical condition which is a stable and static power condition. This slight over percentage of supercriticality would allow for a higher impartment of kinetic energy through heat to a propellant such as diatomic hydrogen which has a very low molecular weight and which is easy to store onboard a spacecraft or can even be collected from interstellar space in sufficient amounts.

The gaseous core reactor itself is made in a cylindrical fashion and in this paper, we take a gaseous core with a length of 7 meters and an opening diameter of 3 meters. The entrance to the reactor is a mixing chamber where Uranium Hexafluoride, as well as Hydrogen, are inserted for mixing. The velocity of the Hydrogen is 100 times faster than Uranium Hexafluoride when inserted into the chamber. Moreover, there are also other mixing constraints as it is essential to mix one molecule of Uranium Hexafluoride for every thousand molecules of hydrogen to conserve nuclear fuel and to achieve an appropriate amount of criticality inside the reactor itself. It is very important to ensure that the mixing of uranium hexafluoride with hydrogen is conducted in a homogenous manner since this will help in maintaining a controllable supercritical nuclear reaction. This way, you can make sure that both the spacecraft propulsion conditions as well as nuclear criticality conditions are obtained [11].

In the mixing chamber, some time and space are given so that relatively even mixing conditions are obtained. The hydrogen itself is at -235 degrees Kelvin and the Uranium Hexafluoride is near room temperature. As a result, multiphase flow occurs at that particular junction, and heat as well as mass transfer takes place at the mixing chamber. Of course, there is also some heat backlash due to radiation as well as convection from the nuclear reaction taking place in the reactor itself.

Once the mixing occurs and relative stability conditions are reached, then the second step in such a reactor would be to mix this content into the gaseous core itself. In the gaseous core as soon as the mixture comes into contact with a neutron source, the criticality conditions are immediately reached and as a result, the fission reaction will immediately start as Uranium Hexafluoride is bombarded with neutrons. As the fission reaction progresses, an average of 2.7 neutrons are produced which makes it a self-sustaining reaction. Due to the nature of the nuclear fuel itself, the condition becomes supercritical in a matter of seconds, as soon as the first contact is achieved [12].

The UF₆ which is already injected into the gaseous nuclear core then undergoes fission by bombardment of high-speed neutrons. As the neutrons hit their target, Uranium Hexafluoride undergoes fission and it is separated into the products below while heat energy is released. As a result, this heat energy is transferred to the H₂ molecules [11].

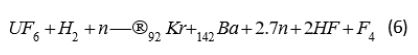
Due to the fact that the nuclear fuel is in gaseous form, there is no need to worry about a fuel rod meltdown as it is in the case of the NTR. Of course, the outer shielding of the reactor core will still need to be strengthened to withstand the temperature range of 5000K to 10000K in order to reach a high specific impulse for a Mars mission. In order to shield the reactor core boundaries, an MHD field can be used to channel the flow away from the boundaries and directly into the nozzle. Some parameters for a gaseous core nuclear reactor that can be used for Lalande 21185 mission are given below [11].

Table 2: Gas Core Reactor Design Parameters.

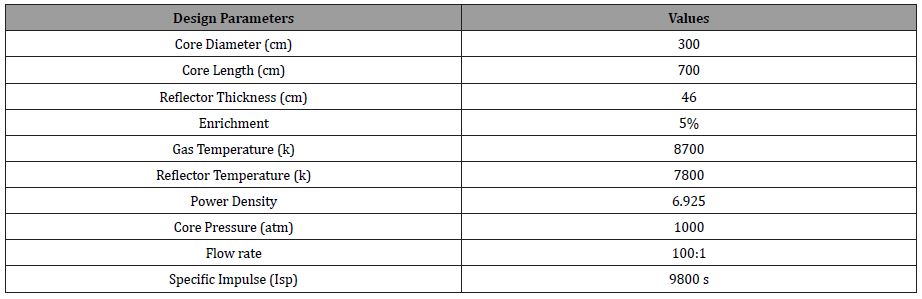
The problem with gaseous core reactors comes from the fact that it is very hard to control the nuclear reaction, since there are no control rods or any scram rods that are found in normal reactors. As a result, the control of the reactor is only done through the changing of pressure or the reduction or increase in the amount of Uranium Hexafluoride that is being pumped into the system. Thus, the criticality of the nuclear reactor itself will need to be matched to the propulsion system input and output in order to reach the desired safety standards and also to make sure that the smooth operation of the propulsion system is carried out. Of course, it is important to consider that spacecraft stability needs to be calculated and factored in since the operation of the reactor may also cause unwanted vibrations in the spacecraft hull, which will need to be dampened so that the spacecraft or the research probe doesn’t spiral out of control at semi-relativistic speeds.

Hence, the specific impulse of the spacecraft can be increased to large amounts by the heat that is being produced by this type of nuclear reactor [12]. The specific impulse can be increased in this type of system by increasing the core diameter of the gaseous core reactor.

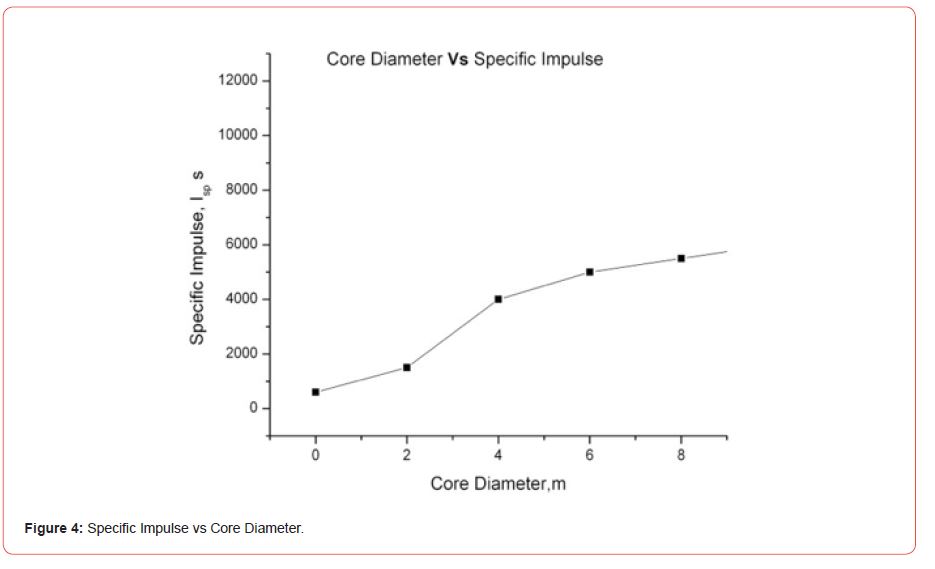
One advantage of using gaseous core reactors would be that they will be able to provide continuous access to the propulsion system of the spacecraft. This way, the required accelerations can be attained.

Of course, more exotic means of spacecraft propulsion such as Fusion drive and Antimatter drive can also be considered for a mission to Lalande 21185 [13]. However, these technologies are beyond our reach at the beginning of the 21st century, while Gaseous core reactor technology would allow mankind to achieve up to 0.4c of acceleration within the means of technology that is available today. Hence, these parameters would allow the planning of such a mission to make interstellar travel a reality for everyone [14].

Based on the above information provided, now it’s possible to take a case study of an interstellar flight to the Lalande 21185 system using a gaseous core nuclear propulsion system where a speed of Ve=0.4c is reached.

By taking an average distance of 8.31 lightyears and a speed of 0.4c with Equation 5:

t*=D/V where D=8.31 light years and V=0.4c where c=299,792,458 meters per second

Let’s solve for t using the given values:

t’ = D / v

t = t’ * √(1 - v²/c²)

= (D / v) * √(1 - v²/c²)

Substituting the values, we have:

t = (8.31 light-years / (0.4 * c)) * √(1 -(0.4c)²/c²)

= (8.31 * 9.461 × 10¹⁵ meters) / (0.4 * 299,792,458 m/s) * √(1 - 0.42) ≈ (7.850 × 10¹⁶ meters) /(119,917,183 m/s) * √(1 - 0.16) ≈ 654,960,943 seconds

Converting seconds to years:

≈ 654,960,943 seconds / (60 seconds * 60 minutes * 24 hours * 365.25 days)≈ 20.75 years

Therefore, it would take approximately 20.75 years to reach the Lalande 21185-star system from Earth traveling at 0.4 times the speed of light (0.4c) with zero acceleration and if the speed was reached instantaneously without any acceleration. This doesn’t take deceleration into account as well. However, it tells us that o.4c, which is an attainable speed with gaseous core nuclear propulsion, would definitely allow an unmanned probe to reach Lalande 21185 in roughly 21 years with today’s technology (with no acceleration and deceleration taken into account).

Of course, for a more realistic calculation, it is essential to take acceleration and deceleration into account. Let’s imagine that we will be accelerating at an acceleration rate of 1g until we reach the speed of 0.4c. Then let’s imagine that the unmanned space probe can continue at the speed of 0.4c with zero acceleration until it comes close to the Lalande 21185-star system. At an appropriate distance let’s imagine a deceleration to a speed of zero from 0.4c with a deceleration rate of 1g to match our acceleration rate.

So, to summarize the journey, from a High Earth Orbit, if we ideally travel in a straight line (which won’t be the case in reality), starting at zero speed and accelerating with an acceleration rate of 1g until the unmanned spacecraft reaches a speed of 0.4c. Once the spacecraft reaches that speed, the speed of 0.4c remains constant and the spacecraft continues unimpeded at that speed until it gets close to the Lalande 21185-star system. At a certain pre-calculated range, the spacecraft would decelerate at

04.g until it reaches zero speed around an orbit of Lalande 21185. Hence, we would need to calculate these to get a more realistic time.

To calculate the time, it takes to reach a speed equal to 40 percent of the speed of light (0.4c) using a constant acceleration of 1g (9.8 meters per second squared), we can use the equations of motion.

Let’s denote the final velocity as v (0.4c) and the acceleration as a (1g or 9.8 m/s²).

The formula to calculate the time it takes to reach a certain velocity from rest with constant acceleration is:

v = u + at (6)

where:

v is the final velocity

u is the initial velocity (0 m/s in this case) a is the acceleration

t is the time

Rearranging the formula to solve for time (t): t = (v - u) / a

Substituting the given values:

t = (0.4c - 0) / (1g)

= (0.4 * 299,792,458 m/s) / (9.8 m/s²)≈ 12,193,829 seconds

Converting seconds to years:

t ≈ 12,193,829 seconds / (60 seconds * 60 minutes * 24 hours * 365.25 days)≈ 0.387 years

Therefore, it would take approximately 0.387 years (or about 4.64 months) to reach a speed equal to 40 percent of the speed of light (0.4c) using a constant acceleration of 1g.

It can be stated that the deceleration from 04.c speed to zero would be calculated in a similar manner and that would also give a similar result of 0.387 years. Hence, the unmanned spacecraft would need to spend 0.774 years for accelerating and decelerating besides the time spent traveling at a constant velocity of 04.c.

Now, if we use simple physics equations of motion, we can calculate the amount of distance traveled from zero velocity and accelerating at 1g until reaching 0.4 light years. This would be 7.16 × 1014 meters, which would be ≈ 0.0757 light-years. The same calculation would hold for the deceleration phase as well with ≈ 0.0757 light-years of distance spent in decelerating for a total of 0.1514 lightyears to accelerate and decelerate. This means that since the distance to Lalande 21185 is 8.31 lightyears, the spacecraft would travel 8.1586 lightyears at a constant speed of 0.4c. With the same calculations shown at the beginning of the section, we would approximately get a time of 20.42 years of traveling at constant velocity.

Thus, finally, we can say that as per time spent on spacecraft, 0.387 years for acceleration(1g), 2.42 years at a constant speed (0.4c), and finally at 0.387 years at deceleration(1g), we would get approximately 21.19 years of spacecraft travel time. Of course, since semi-relativistic speeds are concerned, the time spent on Earth would be longer. It can be said that 21.19 years of travel at sub-light speeds using advanced nuclear propulsion technology is something that can certainly be achieved with 21st-century technology. However, the effects of acceleration and deceleration on the spacecraft would need to be examined, as well as the effects that relativity may have on the fission reaction [15-17].

As stated above, mankind is looking for new and exotic ways to start an interstellar journey to the nearest stars. Even if at the end of the mission, nothing is found, it will at least prove to mankind that travel to the stars is possible. Lalande 21185 represents the culmination of these dreams as mankind can reach even further destinations after such a mission. As seen from the above calculations, approximately 21 years is not such a long mission time considering the fact that the Voyager II space probe has been operational for 46 years as of now, since its launch in 1977 until 2023. Even though it is not possible to reach such a destination yet, this paper gives an overview of the requirements for such a mission in the hopes that it will take its place in the history of spaceflight, so that the dream of stars can be realized someday. It is hoped that this paper and similar papers will pave the way for the future for conducting similar studies, so that these calculations can be matched with a cost analysis to start planning for such missions within the 21^st century.

I would like to acknowledge the help of Prof. Dr. Murat Aydin, Prof. Dr. Akif Atalay, Prof. Dr. Ruhi Kaykayoglu, and Dr. Turgut Berat Karyot, for their past contributions to the conceptual analysis.

None.

1. Deborah Cadbury (2005) Space Race: The Untold Story of Rivals and Their Struggle for the Moon. Fourth Estate.
2. Stanley Schmidt, Robert Zubrin (1996) Islands in The Sky: Bold New Idea Colonizing Space. Wiley, First Edition.
3. Giancarlo Genta, Michael J Rycroft (2003) Space, the final frontier? Cambridge University Press, UK.
4. Russell HN (1905) The Parallax of Lalande 21185. Monthly Notices of the Royal Astronomical Society 65: 787-800, London.
5. Robert Prokop (2012) Observing the Nearest Stars. ISBN 978-1-105-83452-3, Maryland, USA.
6. Ian A Crawford (2011) Project Icarus: A review of Local Interstellar Medium Properties of Relevance for Space Missions to the Nearest Star. Acta Astronautica 68(2011): 691-699.
7. Dana G Andrews (1994) Cost Considerations for Interstellar Missions. Acta Astronautica 34: 357-365.
8. John Allen, Mark Nelson (1999) Overview and Design Biospherics and Biosphere 2, mission one (1991-1993). Ecological Engineering 13: 15-29.
9. A Lyngvi, P Falkner, S Cambel (2005) The Interstellar Heliopause Probe. Acta Astronautica 57: 104-111.
10. R L McNutt, GB Andrew (2004) A Realistic Interstellar Explorer. Advances in Space Research 34: 192-197.
11. Gurunadh Velidi, Ugur Guven (2011) Usage of Nuclear Reactors for Space Applications: Space Propulsion and Space Power Concepts. in proc. International Conference on Mechanical and Aerospace Engineering, New Delhi, P. 356-360.
12. Paul A Czysz, Claudio Bruno (2009) Future Space Craft Propulsion Systems: Enabling Technologies for Space Exploration, Second Edition, Praxis Publishing Ltd, Chichester, UK.
13. Claudio Bruno (2008) Nuclear Space Power and Propulsion Systems. 225: Progresses in Astronautics and Aeronautics, American Institute of Aeronautics and Astronautics.
14. W Huntress, D Setson (2006) The Next Steps in Exploring Deep Space-A Cosmic Study by the IAA. Acta Astronautica 58: 304-377.
15. Yamashiki YA (2019) Impact of Stellar Superflares on Planetary Habitability. The Astrophysical Journal 881: 114.
16. Gatewood GD (1996) Lalande 21185. Bulletin of the American Astronomical Society. 28: 885.
17. Guven U (2012) Interstellar Mission to Lalande 21185 Possibilities for the Future. 63^rd International Astronautical Congress, Naples, Italy, IAC-12-D4,1,7,x15192.